In this world of few certainties, there is one thing that I am certain of: I am not certain how the tides work. Oh, it’s the moon’s gravity, and I think the sun is involved. Well, then, how is it that on a new moon, when the sun and the moon are both on the same side of the earth, we still have two tides? Shouldn’t there be just one big one?

I was reminded of these puzzles recently because of what the news media were calling “King Tides.” This isn’t official terminology from NOAA; it’s used to sensationalize the highest tides of the year. The media routinely explained that these tides occur “when the sun, moon, and earth are in alignment.” But wait just a durned minute. Doesn’t that happen twice in every single month, in which case they are just plain old “spring” tides? How do these get the royal treatment if they’re no different from the commoners?
King Tides occur near perihelion, when the earth is closest—not by all that much—to the sun. Perihelion occurred on January 4. If it got real close, you’d think we’d feel pretty warm. But no, it happens in winter. The highest tide this season was 7.18 feet on December 13, 2012 on a new moon.
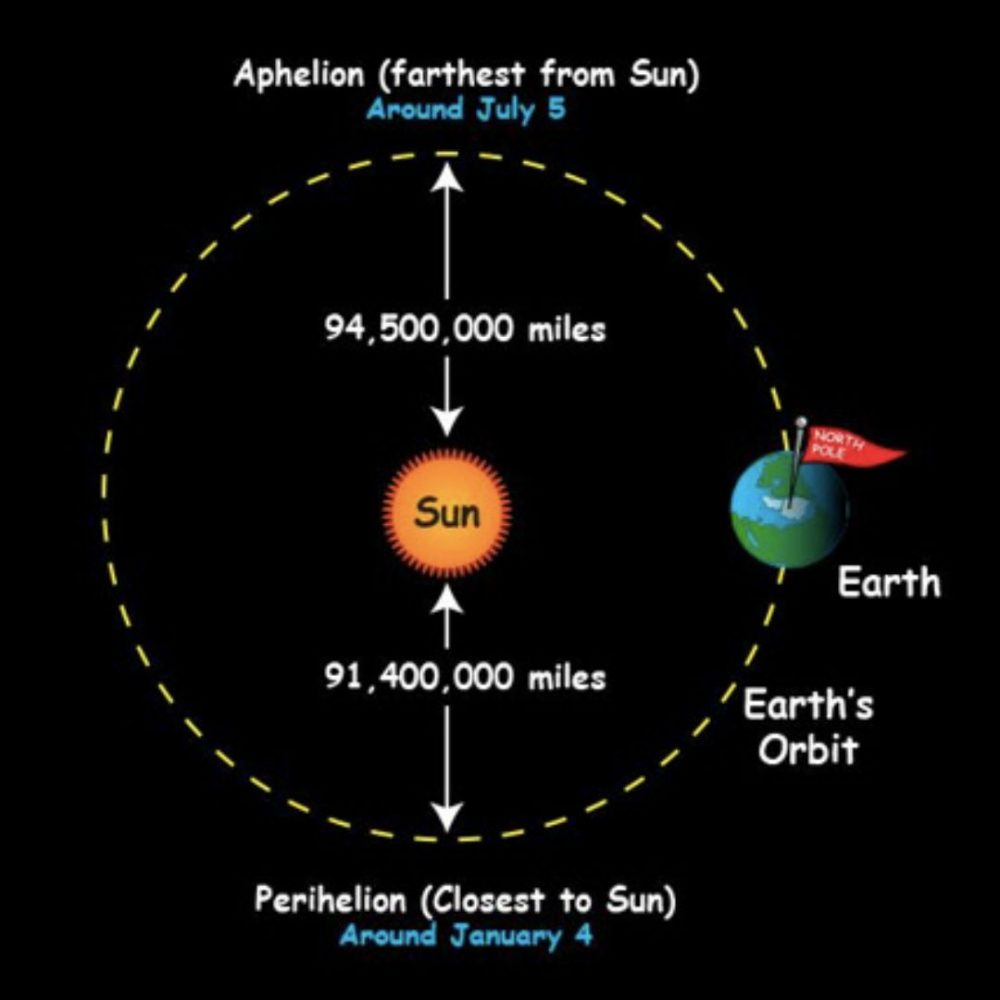
However, six months later, we again have very high tides, when the earth is at aphelion, its farthest from the sun, and paradoxically, it is hot. On July 21, on a full moon, the predicted tide is 7.07 feet, barely lower than the December tide. So our distance from the sun isn’t the only factor. Since this is a yearly cycle, it is the result of the sun’s influence, not the moon’s monthly pattern. It turns out the latitude of the sun is as important as its distance, and at our summer solstice the sun’s over the northern hemisphere. This makes up for the greater distance and generates our big summer tides. In Australia, where, as best I can figure, The “King” tides got their name, summer solstice occurs during perihelion, meaning latitude and distance are both at their peak influence. So if you want to experience the whole shebang, you’ll have to go down under.
But it’s nowhere near this simple. Again, of this I’m certain. We used three tidal constituents for this analysis. NOAA uses 37, but the total number is 396.
